Name:_______________________
Test 3
05/23/2006
Ch. 23-26
Useful number(s): c=3.00x108 m/s, h=6.626x10-34
J·s
1)
An object 8cm tall is
placed 15cm in front of a mirror (f=-100mm).
a)
Draw a diagram of this
situation, including 2 rays, which originate from the top of the object and
terminate on the top of the image.
b)
Is the mirror concave
or convex?
c)
How far is the center
of curvature from the mirror?
d)
What are the height,
position, and orientation of the image?
e)
Is the image real or
virtual?
Name:_______________________
2)
You would like to know
the focal length of your camera lens.
So you set up an experiment.
You place a meter stick 1m away from the center of the camera lens and
take a picture. You then develop the
film and measure the height of the image on the film to be 25.60mm. (Hint: you can assume that the lens is
converging and the image is inverted.)
a)
What is the focal
length of the lens?
b)
What is the distance
from the lens to the image?
Name:_______________________
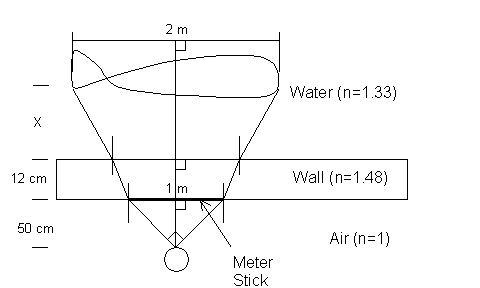
3)
A 2m long fish is
hovering directly in front of you in a large aquarium filled with water
(n=1.33). The wall of the aquarium is
12cm thick with n=1.48. A horizontal
meter stick is attached to air-side of the wall. When you stand 50cm in front of the wall with the center of the
meter stick and the center of the fish directly in front of your eyes, the
meter stick and the fish appear to be the same length. (See the diagram below. NB: Not drawn to scale) How far is the fish
from the water-side of the wall. (i.e.,
the distance labeled X in the diagram.)
Name:_______________________
4)
In the following circuit: 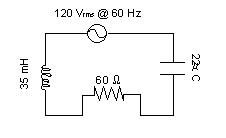
a) (9 pts.) What is the power in each element?
b) (3 pts.) Does the total current lead or lag the total
voltage?
c) (4 pts.) What is the resonance frequency of the
circuit?
d) (4 pts.) What would the power dissipated by the whole
circuit if the frequency was changed to the resonance frequency?
Name:_______________________
5)
A light ray traveling
in air (n=1.00) hits a 2m by 3m rectangular piece of glass in the center of the
short side at an incident angle of 55 degrees.
The ray enters the glass and reflects off of the top surface and exits
through the center of the opposite short side.
a)
What is the index of
refraction of the glass?
b)
What is the critical
angle for internal reflection for the ray inside the glass?
c)
What angle (with
respect to the normal) does the ray hit the top surface of the glass?
d)
Are your answers to
parts b) and c) consistent with the fact that the light is reflected at the top
surface? Explain.
e)
The air is replaced by
water (n=1.33), but the ray still initially hits the same glass at the center
of the short side at angle of incident of 55 degrees.
i)
From what side of the
glass will the ray emerge?
ii)
At what angle with
respect to the normal will it emerge?